Next: MC342 History of mathematics
Up: Year 3
Previous: MC320 Modelling Biological Systems
MC341 Group Theory
| Credits: 20 |
Convenor: Dr. R. Marsh |
Semester: 2 |
| Prerequisites: |
essential: MC242 |
|
| Assessment: |
Coursework: 10% |
Three hour exam: 90% |
| Lectures: |
36 |
Classes: |
10 |
| Tutorials: |
none |
Private Study: |
104 |
| Labs: |
none |
Seminars: |
none |
| Project: |
none |
Other: |
none |
| Total: |
150 |
|
|
Explanation of Pre-requisites
Although a brief revision of the material from MC242 will take place
at the start of the course, it is essential that students are
familiar with the content of that course.
Course Description
This module builds upon MC242 (Introduction to Groups) and gives an overview
of the main ideas of group theory. The emphasis is on structure theorems,
classification results and decomposition concepts that have evolved as a result
of attempts to describe all possible groups. Although such a description is not
actually feasible, it is possible to obtain surprisingly detailed information
about the structure of large classes of groups.
One of the major goals of the module is to develop enough theory to be able to
discuss the classification of the finite simple groups, at least in broad general
terms. This classification ranks as one of the major achievements in pure
mathematics. Its proof runs to somewhere between 10,000 and 15,000 journal
pages, spread across some 500 separate articles by more than 100 mathematicians,
almost all written between 1950 and the early 1980's. A revision is currently
underway, but even this is expected to run to more than 5,000 pages.
The module is designed to present a broad outline of the classification, to
explain its significance, and to give a hint of the complexity of its proof.
Aims
This course aims to present the fundamental ideas of group theory by studying
the structure theorems and decomposition concepts that arise in attempts to
understand groups in terms of less complicated groups. These attempts are
most successful in studying finite groups because there is a sense in which
any finite group can be regarded as a group built from finite simple groups.
The course aims to develop the ideas necessary to make this notion precise
and to develop the theory needed to present a rough idea of the statement of
the classification of finite simple groups.
Objectives
By the end of this module students should have developed an understanding of
- the concepts of normal subgroups and quotient groups and their relevance
to the structure of a group;
- the idea of a group action and how group actions are used to prove
fundamental results such as the Sylow theorems;
- the idea of a group presentation and how to calculate using generators
and relations;
- the properties of permutations and of symmetric and alternating groups;
- the idea of simple groups as the basic building blocks of group theory.
Transferable Skills
This module should help the student develop a good sense of the axiomatic
approach to mathematics. In addition, it provides students with practice in
presenting reasoned arguments with precision and cogency.
Syllabus
Definition and examples of groups; direct products; permutations and
symmetric groups; equivalence relations; free groups; subgroups; products
of subsets; cosets and Lagrange's Theorem
Conjugates and conjugacy classes of elements and of subsets; normal subgroups;
quotient groups; centralisers and normalisers; the centre of a group; normal
closures; homomorphisms and isomorphisms; kernels and images; the isomorphism
theorems; the alternating groups An; automorphism groups and the conjugation map;
generators and relations
G-sets and actions; the kernel of an action; faithful and transitive actions;
multiplication and conjugation actions; stabilisers and orbits; the
Orbit-Stabiliser Theorem; Sylow subgroups of finite groups; the Sylow theorems;
characteristic subgroups
Simple groups; normal and subnormal series; composition series and chief
series; the Jordan-Hölder Theorem; simplicity of the alternating groups
An for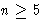 
; solvable and nilpotent groups; commutator subgroups;
upper and lower central series; derived series; outline of the classification
of finite simple groups
Reading list
Recommended:
J. F. Humphreys,
A Course in Group Theory,
Oxford University Press.
Background:
J. A. Gallian,
Contemporary Abstract Algebra,
DC Heath.
D. L. Johnson,
Presentations of Groups,
Cambridge University Press.
C. R. Jordan and D. A. Jordan,
Groups,
Edward Arnold.
I. D. MacDonald,
Theory of Groups,
Oxford University Press.
J. S. Rose,
A Course in Group Theory,
Cambridge University Pres.
J.J. Rotman,
An Introduction to the Theory of Groups,
Springer-Verlag.
Details of Assessment
Coursework consists of approximately seven homework assignments handed out
periodically during the semester. This coursework contributes 10% toward
the assessment, and the final examination contributes the remaining 90%.
Students have three hours to complete the final examination and can obtain
full marks by correctly answering five out of eight questions, all of which
have equal weight. Calculators are not allowed.
Next: MC342 History of mathematics
Up: Year 3
Previous: MC320 Modelling Biological Systems
S. J. Ambler
11/20/1999