


Next: MC225 Dynamics: orbits and
Up: Year 2
Previous: MC223 Relativity
MC224 Vector Calculus
| Credits: 10 |
Convenor: Dr. M. Walmsley |
Semester: 1 (weeks 7 to 12) |
| Prerequisites: |
essential: MC120, MC121 |
desirable: MC144, MC145, MC146, MC147, MC248 |
| Assessment: |
Coursework: 20% |
One and a half hour exam: 80% |
| Lectures: |
18 |
Classes: |
6 |
| Tutorials: |
6 |
Private Study: |
51 |
| Labs: |
none |
Seminars: |
none |
| Project: |
none |
Other: |
none |
| Total: |
75 |
|
|
Explanation of Pre-requisites
A knowledge of Vector Algebra from MC120 is assumed and only one lecture
will be spent on its revision. Also required is a knowledge of partial
differentiation (MC121 or elsewhere) and a general mathematical knowledge
from other modules.
Course Description
This module will extend the vector algebra of the first year to the calculus
of three dimensional vectors. This is an essential module for those wishing
to take certain later modules in Applied Mathematics, for example General
Relativity, Electromagnetic Theory etc.
Aims
The use of vectors simplifies and condenses the
mathematical discussion of many problems which arise in applied mathematics
and so this module forms a basis for many later modules. However, vector
calculus may be studied in its own right and here links with multivariable
analysis will be apparent.
Objectives
To provide a working knowledge of vector calculus upto and including Stokes'
Theorem and the Divergence Theorem. To provide an introduction to the
summation convention providing a background for later work with cartesian
tensors.
Transferable Skills
This module provides essential mathematics for any practising applied
mathematician.
Syllabus
Brief revision of vector algebra and introduction/revision of partial differentiation.
Introduction of suffix notation and the summation convention including
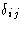 and
and 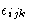 .
.
The vector differential operators grad, div and curl.
Line, surface and volume integrals with particular application to the
divergence theorem and Stokes' theorem.
Reading list
Background:
M. R. Spiegel,
Vector Analysis,
Schaum Outline Series.
H. P. Hsu,
Applied Vector Calculus,
Harcourt Brace Jovanovich College Outline Series.
E. A. Maxwell,
Coordinate Geometry with Vectors and Tensors,
CUP? Probably out of print.
There are in addition a number of Vector Analysis texts located at 515.63 in
the Library.



Next: MC225 Dynamics: orbits and
Up: Year 2
Previous: MC223 Relativity
Roy L. Crole
10/22/1998
![]() and
and ![]() .
.