| Credits: 10 | Convenor: Dr. J.F. Watters | Semester: 1 (weeks 7 to 12) |
| Prerequisites: | essential: MC144, MC145, MC147 | |
| Assessment: | Coursework: 20% | One and a half hour exam in January: 80% |
| Lectures: | 18 | Classes: | 5 |
| Tutorials: | none | Private Study: | 52 |
| Labs: | none | Seminars: | none |
| Project: | none | Other: | none |
| Total: | 75 |
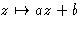. The extended complex plane
(inversive plane) is defined
and properties of bilinear transformations, e.g.
mappings of the sort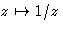 
, are explored using
stereographic projection. The final topic is a brief discussion of the affine
plane using matrices and here the basic idea is to examine mappings of the
plane which are required simply to map lines to lines.
To construct transformations with specific properties.
To be familiar with some geometric invariants of groups of transformations of the plane.
To understand stereographic projection and its mapping properties.
To have an understanding of bilinear transformations of the extended complex plane.
To use transformations to solve geometric problems.
To be able to identify the affine nature of a conic.
Developing understanding of mappings and some group-theoretical ideas.
Transformation as a problem solving technique.
Written presentation of logical argument in geometric settings.
Isometries as transformations of the complex plane; products of reflections; properties invariant under isometries; classification of isometries. Apply isometric transformations to solve geometric construction problems.
Similarities as transformations of the complex plane; properties invariant under similarities; classification of similarities. Apply similarity transformations to solve geometric construction problems. Define and recognise groups of similarities (isometries).
Define inversion and bilinear transformations of the complex plane; stereographic projection and the extended complex plane; properties invariant under bilinear transformations (e.g. cross-ratio). Apply inversion to solve geometric construction problems.
Define affine transformations of the plane; properties invariant under affine transformations (e.g. ratio). Obtain affine equivalent forms of conics.
H. S. M. Coxeter, Introduction to Geometry, 2nd edition, Wiley.
M. Jeger, Transformation Geometry, Allen and Unwin.
P. J. Ryan, Euclidean and Non-Euclidean Geometry - an Analytic Approach, Cambridge University Press.
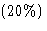.Examination - one and a half hours duration with four questions, all of equal
weight, three to
be answered for full marks.